Aristotelian physics

Aristotelian Physics the natural sciences, are described in the works of the Greek philosopher Aristotle (384 BC – 322 BC). In the Physics, Aristotle established general principles of change that govern all natural bodies; both living and inanimate, celestial and terrestrial—including all motion, change in respect to place, change in respect to size or number, qualitative change of any kind, and coming to be and passing away. As Martin Heidegger, one of the foremost philosophers of the twentieth century, once wrote,

Aristotelian "physics" is different from what we mean today by this word, not only to the extent that it belongs to antiquity whereas the modern physical sciences belong to modernity, rather above all it is different by virtue of the fact that Aristotle's "physics" is philosophy, whereas modern physics is a positive science that presupposes a philosophy.... This book determines the warp and woof of the whole of Western thinking, even at that place where it, as modern thinking, appears to think at odds with ancient thinking. But opposition is invariably comprised of a decisive, and often even perilous, dependence. Without Aristotle's Physics there would have been no Galileo.[1]

To Aristotle, physics is a broad term that includes all nature sciences, such as philosophy of mind, body, sensory experience, memory and biology, and constitutes the foundational thinking underlying many of his works.

Contents

Ancient concepts

Some concepts involved in Aristotle's physics are:

  1. Teleology: Aristotle observes that natural things tend toward definite goals or ends insofar as they are natural. Regularities manifest a rudimentary kind of teleology.
  2. Natural motion: Terrestrial objects tend toward a different part of the universe according to their composition of the four elements. For example, earth, the heaviest element, tends toward the center of the universe—hence the reason for the Earth being at the center. At the opposite extreme the lightest element, fire, tends upward, away from the center. The relative proportion of the four elements composing an object determines its motion. The elements are not proper substances in Aristotelian theory or the modern sense of the word. Refining an arbitrarily pure sample of an element isn't possible; They were abstractions; one might consider an arbitrarily pure sample of a terrestrial substance having a large ratio of one element relative to the others.
  3. Terrestrial motion: Terrestrial objects move downward or upward toward their natural place. Motion from side to side results from the turbulent collision and sliding of the objects as well as transformations between the elements, (generation and corruption).
  4. Rectilinear motion: Ideal terrestrial motion would proceed straight up or straight down at constant speed. Celestial motion is always ideal, it is circular and its speed is constant.
  5. Speed, weight and resistance: The ideal speed of a terrestrial object is directly proportional to its weight. In nature, however, the matter obstructing an object's path is a limiting factor that's inversely proportional to the viscosity of the medium.
  6. Vacuum isn't possible: Vacuum doesn't occur, but hypothetically, terrestrial motion in a vacuum would be indefinitely fast.
  7. Continuum: Aristotle argues against the indivisibles of Democritus (which differ considerably from the historical and the modern use of the term atom).
  8. Aether: The "greater and lesser lights of heaven", (the sun, moon, planets and stars), are embedded in perfectly concentric crystal spheres that rotate eternally at fixed rates. Because the spheres never change and (meteorites notwithstanding) don't fall down or rise up from the ground, they cannot be composed of the four terrestrial elements. Much as Homer's æthere (αἰθήρ), the "pure air" of Mount Olympus was the divine counterpart of the air (άήρ, aer) breathed by mortals, the celestial spheres are composed of a special element, eternal and unchanging, with circular natural motion.
  9. Terrestrial change: Unlike the eternal and unchanging celestial aether, each of the four terrestrial elements are capable of changing into either of the two elements they share a property with: e.g. the cold and wet (water) can transform into the hot and wet (air) or the cold and dry (earth) and any apparent change into the hot and dry (fire) is actually a two step process. These properties are predicated of an actual substance relative to the work it's able to do; that of heating or chilling and of desiccating or moistening. The four elements exist only with regard to this capacity and relative to some potential work. The celestial element is eternal and unchanging, so only the four terrestrial elements account for coming to be and passing away; also called "generation and corruption" after the Latin title of Aristotle's De Generatione et Corruptione (Περὶ γενέσεως καὶ φθορᾶ).
  10. Celestial motion: The crystal spheres carrying the sun, moon and stars move eternally with unchanging circular motion. They're composed of solid aether and no gaps exist between the spheres. Spheres are embedded within spheres to account for the wandering stars, (i.e. the modern planets, which appear to move erratically in comparison to the sun, moon and stars). Later, the belief that all spheres are concentric was forsaken in favor of Ptolemy's deferent and epicycle. Aristotle submits to the calculations of astronomers regarding the total number of spheres and various accounts give a number in the neighborhood of 50 spheres. An unmoved mover is assumed for each sphere, including a prime mover for the sphere of fixed stars. The unmoved movers do not push the spheres (nor could they, they're insubstantial and dimensionless); rather, they're the final cause of the motion, meaning they explain it in a way that's similar to the explanation "the soul is moved by beauty". They simply "think about thinking", eternally without change, which is the idea of "being qua being" in Aristotle reformulation of Plato's theory.

While consistent with common human experience, Aristotle's principles were not based on controlled, quantitative experiments, so, while they account for many broad features of nature, they do not describe our universe in the precise, quantitative way we have more recently come to expect from science. Contemporaries of Aristotle like Aristarchus rejected these principles in favor of heliocentrism, but their ideas were not widely accepted. Aristotle's principles were difficult to disprove merely through casual everyday observation, but later development of the scientific method challenged his views with experiments, careful measurement, and more advanced technology such as the telescope and vacuum pump.

Elements

Aristotle taught that the elements which compose the Earth are different from the one that composes the heavens.[2] He believed that four elements make up everything under the moon (the terrestrial): earth, air, fire and water.[a][3] He also held that the heavens are made of a special, fifth element called "aether",[3] which is weightless and "incorruptible" (which is to say, it doesn't change).[3] Aether is also known by the name "quintessence"—literally, "fifth substance".[4]

He considered heavy substances such as iron and other metals to consist primarily of the element earth, with a smaller amount of the other three terrestrial elements. Other, lighter objects, he believed, have less earth, relative to the other three elements in their composition.[4]

Motion

Aristotle held that each of the four terrestrial (or worldly) elements move toward their natural place, and that this natural motion would proceed unless hindered. For instance, because smoke is mainly air, it rises toward the sky but not as high as fire. He also taught that objects move against their natural motion only when forced (i.e. pushed) in a different direction and only while that force is being applied.[2] This idea had flaws that were apparent to Aristotle and his contemporaries. It was questionable, for example, how an arrow would continue to fly forward after leaving the bowstring; which could no longer be forcing it forward. In response, Aristotle suggested the air behind an arrow in flight is thinned and the surrounding air, rushing in to fill that potential vacuum, is what pushes it forward.[2] This was consistent with his explanation of a medium, such as air or water, causing resistance to the motion of an object passing through it. The turbulent motion of air around an arrow in flight is very complicated, and still not fully understood.

A vacuum, or void, is a place free of everything, and Aristotle argued against the possibility. Aristotle believed that the speed of an object's motion is proportional to the force being applied (or the object's weight in the case of natural motion) and inversely proportional to the viscosity of the medium; the more tenuous a medium is, the faster the motion. He reasoned that objects moving in a void, could move indefinitely fast and thus, the objects surrounding a void would immediately fill it before it could actually form.[5]

Natural place

The Aristotelian explanation of gravity is that all bodies move toward their natural place. For the element earth, that place is the center of the (geocentric) universe, next comes the natural place of water (in a concentric shell around that of earth). The natural place of air is likewise a concentric shell surrounding the place of water. Sea level is between those two. Finally, the natural place of fire is higher than that of air but below the innermost celestial sphere, (the one carrying the Moon). Even at locations well above sea level, such as a mountain top, an object made mostly of the former two elements tends to fall and objects made mostly of the latter two tend to rise.

Medieval commentary

Main article: Theory of impetus

The Aristotelian theory of motion came under criticism and/or modification during the Middle Ages. The first such modification came from John Philoponus in the 6th century. He partly accepted Aristotle's theory that "continuation of motion depends on continued action of a force," but modified it to include his idea that the hurled body acquires a motive power or inclination for forced movement from the agent producing the initial motion and that this power secures the continuation of such motion. However, he argued that this impressed virtue was temporary; that it was a self-expending inclination, and thus the violent motion produced comes to an end, changing back into natural motion. In the 11th century, the Persian polymath Avicenna, in The Book of Healing (1027) was influenced by Philoponus' theory in its rough outline, but took it much further to present the first alternative to the Aristotelian theory. In the Avicennan theory of motion, the violent inclination he conceived was non-self-consuming, a permanent force whose effect was dissipated only as a result of external agents such as air resistance, making him "the first to conceive such a permanent type of impressed virtue for non-natural motion." Such a self-motion (mayl) is "almost the opposite of the Aristotelian conception of violent motion of the projectile type, and it is rather reminiscent of the principle of inertia, i.e., Newton's first law of motion."[6]

The eldest Banū Mūsā brother, Ja'far Muhammad ibn Mūsā ibn Shākir (800-873), wrote the Astral Motion and The Force of Attraction. The Persian physicist, Ibn al-Haytham (965-1039), discussed the theory of attraction between bodies. It seems that he was aware of the magnitude of acceleration due to gravity and he discovered that the heavenly bodies "were accountable to the laws of physics".[7] The Persian polymath Abū Rayhān al-Bīrūnī (973-1048) was the first to realize that acceleration is connected with non-uniform motion, part of Newton's second law of motion. [8] During his debate with Avicenna, al-Biruni also criticized the Aristotelian theory of gravity for denying the existence of levity or gravity in the celestial spheres and for its notion of circular motion being an innate property of the heavenly bodies.[9]

In 1121, al-Khazini, in The Book of the Balance of Wisdom, proposed that the gravity and gravitational potential energy of a body varies depending on its distance from the centre of the Earth.[10] Hibat Allah Abu'l-Barakat al-Baghdaadi (1080–1165) wrote a critique of Aristotelian physics entitled al-Mu'tabar, where he negated Aristotle's idea that a constant force produces uniform motion, as he realized that a force applied continuously produces acceleration, a fundamental law of classical mechanics and an early foreshadowing of Newton's second law of motion.[11] Like Newton, he described acceleration as the rate of change of speed.[12]

In the 14th century, Jean Buridan developed the theory of impetus as an alternative to the Aristotelian theory of motion. The theory of impetus was a precursor to the concepts of inertia and momentum in classical mechanics.[13] Buridan and Albert of Saxony also refer to Abu'l-Barakat in explaining that the acceleration of a falling body is a result of its increasing impetus.[14] In the 16th century, Al-Birjandi discussed the possibility of the Earth's rotation. In his analysis of what might occur if the Earth were rotating, he developed a hypothesis similar to Galileo Galilei's notion of "circular inertia",[15] which he described in the following observational test:

"The small or large rock will fall to the Earth along the path of a line that is perpendicular to the plane (sath) of the horizon; this is witnessed by experience (tajriba). And this perpendicular is away from the tangent point of the Earth’s sphere and the plane of the perceived (hissi) horizon. This point moves with the motion of the Earth and thus there will be no difference in place of fall of the two rocks."[16]

Life and death of Aristotelian physics

The reign of Aristotelian physics lasted for almost two millennia, and provide the earliest known speculative theories of physics. After the work of Galileo, Descartes, and many others, it became generally accepted that Aristotelian physics were not correct or viable.[4] Despite this, scholastic science survived into the late seventeenth century, (perhaps later), when universities amended their curricula.

In Europe, Aristotle's theory was first convincingly discredited by the work of Galileo Galilei. Using a telescope, Galileo observed that the moon was not entirely smooth, but had craters and mountains, contradicting the Aristotelian idea of an incorruptible perfectly smooth moon. Galileo also criticized this notion theoretically – a perfectly smooth moon would reflect light unevenly like a shiny billiard ball, so that the edges of the moon's disk would have a different brightness than the point where a tangent plane reflects sunlight directly to the eye. A rough moon reflects in all directions equally, leading to a disk of approximately equal brightness which is what is observed.[17] Galileo also observed that Jupiter has moons, objects which revolve around a body other than the Earth. He noted the phases of Venus, convincingly demonstrating that Venus, and by implication Mercury, travels around the sun, not the Earth.

According to legend, Galileo dropped balls of various densities from the Tower of Pisa and found that lighter and heavier ones fell at almost the same speed. In fact, he did quantitative experiments with balls rolling down an inclined plane, a form of falling that is slow enough to be measured without advanced instruments.

A heavier body falls faster than a lighter one of the same shape in a dense medium like water, and this led Aristotle to speculate that the rate of falling is proportional to the weight and inversely proportional to the density of the medium. From his experience with objects falling in water, he concluded that water is approximately ten times denser than air. By weighing a volume of compressed air, Galileo showed that this overestimates the density of air by a factor of forty.[18] From his experiments with inclined planes, he concluded that all bodies fall at the same rate neglecting friction.

Galileo also advanced a theoretical argument to support his conclusion. He asked if two bodies of different weights and different rates of fall are tied by a string, does the combined system fall faster because it is now more massive, or does the lighter body in its slower fall hold back the heavier body? The only convincing answer is neither: all the systems fall at the same rate.[17]

Followers of Aristotle were aware that the motion of falling bodies was not uniform, but picked up speed with time. Since time is an abstract quantity, the peripatetics postulated that the speed was proportional to the distance. Galileo established experimentally that the speed is proportional to the time, but he also gave a theoretical argument that the speed could not possibly be proportional to the distance. In modern terms, if the rate of fall is proportional to the distance, the differential equation for the distance y travelled after time t is

{dy\over dt} = y

with the condition that 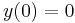y(0)=0. Galileo demonstrated that this system would stay at 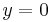y=0 for all time. If a perturbation set the system into motion somehow, the object would pick up speed exponentially in time, not quadratically.[18]

Standing on the surface of the moon in 1971, David Scott famously repeated Galileo's experiment by dropping a feather and a hammer from each hand at the same time. In the absence of a substantial atmosphere, the two objects fell and hit the moon's surface at the same time.

With his law of universal gravitation Isaac Newton was the first to mathematically codify a correct theory of gravity. In this theory, any mass is attracted to any other mass by a force which decreases as the inverse square of their distance. In 1915, Newton's theory was replaced by Albert Einstein's general theory of relativity. See gravity for a much more detailed complete discussion.

See also

Disputed works are marked by *, and ** marks a work generally agreed to be spurious.

Notes

a ^ The term "Earth" does not refer to planet Earth, which is known by modern science to be composed of a large number of chemical elements. Modern chemical elements are not conceptually similar to Aristotle's elements. The term "Air" does not refer to the breathable air. The Earth's atmosphere is also made up of many chemical elements.

References

  1. ^ Martin Heidegger, The Principle of Reason, trans. Reginald Lilly, (Indiana University Press, 1991), 62-63.
  2. ^ a b c "Physics of Aristotle vs. The Physics of Galileo". http://csep10.phys.utk.edu/astr161/lect/history/aristotle_dynamics.html. Retrieved 6 April 2009. 
  3. ^ a b c "www.hep.fsu.edu" (PDF). http://www.hep.fsu.edu/~wahl/Quarknet/pepperlect/aristogalnewt.pdf. Retrieved 26 March 2007. 
  4. ^ a b c "Aristotle's physics". http://aether.lbl.gov/www/classes/p10/aristotle-physics.html. Retrieved 6 April 2009. 
  5. ^ Land, Helen The Order of Nature in Aristotle's Physics: Place and the Elements (1998)
  6. ^ Aydin Sayili (1987), "Ibn Sīnā and Buridan on the Motion of the Projectile", Annals of the New York Academy of Sciences 500 (1): 477–482 [477]:
    According to Aristotle, continuation of motion depends on continued action of a force. The motion of a hurled body, therefore, requires elucidation. Aristotle maintained that the air of the atmosphere was responsible for the continuation of such motion. John Philoponos of the 6th century rejected this Aristotelian view. He claimed that the hurled body acquires a motive power or an inclination for forced movement from the agent producing the initial motion and that this power or condition and not the ambient medium secures the continuation of such motion. According to Philoponos this impressed virtue was temporary. It was a self-expending inclination, and thus the violent motion thus produced comes to an end and changes into natural motion. Ibn Sina adopted this idea in its rough outline, but the violent inclination as he conceived it was a non-self-consuming one. It was a permanent force whose effect got dissipated only as a result of external agents such as air resistance. He is apparently the first to conceive such a permanent type of impressed virtue for non-natural motion. [...] Indeed, self-motion of the type conceived by Ibn Sina is almost the opposite of the Aristotelian conception of violent motion of the projectile type, and it is rather reminiscent of the principle of inertia, i.e., Newton's first law of motion.
  7. ^ Duhem, Pierre (1908, 1969). To Save the Phenomena: An Essay on the Idea of Physical theory from Plato to Galileo, p. 28. University of Chicago Press, Chicago.
  8. ^ O'Connor, John J.; Robertson, Edmund F., "Al-Biruni", MacTutor History of Mathematics archive, University of St Andrews, http://www-history.mcs.st-andrews.ac.uk/Biographies/Al-Biruni.html .
  9. ^ Rafik Berjak and Muzaffar Iqbal, "Ibn Sina--Al-Biruni correspondence", Islam & Science, June 2003.
  10. ^ Mariam Rozhanskaya and I. S. Levinova (1996), "Statics", in Roshdi Rashed, ed., Encyclopedia of the History of Arabic Science, Vol. 2, p. 614-642 [621-622]. Routledge, London and New York.
  11. ^ Shlomo Pines (1970). "Abu'l-Barakāt al-Baghdādī , Hibat Allah". Dictionary of Scientific Biography. 1. New York: Charles Scribner's Sons. pp. 26–28. ISBN 0684101149. 
    (cf. Abel B. Franco (October 2003). "Avempace, Projectile Motion, and Impetus Theory", Journal of the History of Ideas 64 (4), p. 521-546 [528].)
  12. ^ A. C. Crombie, Augustine to Galileo 2, p. 67.
  13. ^ Aydin Sayili (1987), "Ibn Sīnā and Buridan on the Motion of the Projectile", Annals of the New York Academy of Sciences 500 (1): 477–482
  14. ^ Gutman, Oliver (2003). Pseudo-Avicenna, Liber Celi Et Mundi: A Critical Edition. Brill Publishers. p. 193. ISBN 9004132287 
  15. ^ (Ragep 2001b, pp. 63–4)
  16. ^ (Ragep 2001a, pp. 152–3)
  17. ^ a b Galileo Galilei, Dialogue Concerning the Two Chief World Systems.
  18. ^ a b Galileo Galilei, Two New Sciences.

Further Reading